Automatic regulation of the oil level in the pressure t
Content: Doc1.docx (79.77 KB)
Uploaded: 03.09.2019
Positive responses: 0
Negative responses: 0
Sold: 1
Refunds: 0
$0.5
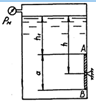
Automatic regulation of the oil level in the pressure tank is carried out by a rotating shield AB (Fig. 18). Find the depth h of immersion of the axis of rotation of the shield and the strength of the hydrostatic pressure of oil on it, if the dimensions of the shield are bx = 1.2x0.6 m, depth h1 and the manometric pressure on the surface of the oil are pM. Build a diagram of hydrostatic pressure.
Given: bha = 1.2x0.6 m; h1 = 3.5m; pM = 50kPa = 50 * 103Pa
Decision
1. We show in Fig. 1 the design scheme of the tank and shield.
Figure 1 - Design scheme
2. Determine the piezometric height in the vessel:
(one)
where p0 is the excess pressure above the surface of the liquid in the vessel; - oil density.
Pressure above the liquid surface
The density of oil is ρ = 900 kg / m3.
Since, the piezometric plane is located above the surface of the liquid.
3. Find the strength of the hydrostatic pressure of the fluid on the shield:
(2)
where is the w-area of the shield; - excess oil pressure on the shield in the center of gravity of its area - point C.
Shield area
(3)
where a and b are the height and width of the shield, respectively
Excessive oil pressure on the shield in the center of gravity of its area
(four)
where hc is the distance from the surface of the liquid to the center of gravity of the cap.
(five)
where h1 is the distance from the surface of the liquid to the edge of the shield.
So, the force of the hydrostatic pressure of the fluid on the shield
4. Find the depth h of immersion of the axis of rotation of the shield. The axis O of rotation of the shield should be at the point of application of the resultant pressure force on the shield under specified conditions, i.e. in the center of pressure. If the oil level exceeds a predetermined one, then the point of application of the resultant force will shift above point O and the shield will turn clockwise as shown in Fig. 1.
Depth h is determined from the expression
(6)
where e is the eccentricity of the application of force R.
We determine the eccentricity of the point of application of force P (see Figure 1) by the formula:
(7)
where is the J0 moment of inertia of the shield relative to the central axis.
Because for a rectangular shield, then the formula (7) takes the form:
(eight)
5. We construct in Fig. 1 diagram of hydrostatic pressure on shield AB.
Absolute oil pressure on the free surface
(9)
Absolute oil pressure at point A of the cap
(ten)
Absolute oil pressure at point B of the cap
(eleven)
Buy and download a ready-made solution.
Product Content: task solution in Word format.
Given: bha = 1.2x0.6 m; h1 = 3.5m; pM = 50kPa = 50 * 103Pa
Decision
1. We show in Fig. 1 the design scheme of the tank and shield.
Figure 1 - Design scheme
2. Determine the piezometric height in the vessel:
(one)
where p0 is the excess pressure above the surface of the liquid in the vessel; - oil density.
Pressure above the liquid surface
The density of oil is ρ = 900 kg / m3.
Since, the piezometric plane is located above the surface of the liquid.
3. Find the strength of the hydrostatic pressure of the fluid on the shield:
(2)
where is the w-area of the shield; - excess oil pressure on the shield in the center of gravity of its area - point C.
Shield area
(3)
where a and b are the height and width of the shield, respectively
Excessive oil pressure on the shield in the center of gravity of its area
(four)
where hc is the distance from the surface of the liquid to the center of gravity of the cap.
(five)
where h1 is the distance from the surface of the liquid to the edge of the shield.
So, the force of the hydrostatic pressure of the fluid on the shield
4. Find the depth h of immersion of the axis of rotation of the shield. The axis O of rotation of the shield should be at the point of application of the resultant pressure force on the shield under specified conditions, i.e. in the center of pressure. If the oil level exceeds a predetermined one, then the point of application of the resultant force will shift above point O and the shield will turn clockwise as shown in Fig. 1.
Depth h is determined from the expression
(6)
where e is the eccentricity of the application of force R.
We determine the eccentricity of the point of application of force P (see Figure 1) by the formula:
(7)
where is the J0 moment of inertia of the shield relative to the central axis.
Because for a rectangular shield, then the formula (7) takes the form:
(eight)
5. We construct in Fig. 1 diagram of hydrostatic pressure on shield AB.
Absolute oil pressure on the free surface
(9)
Absolute oil pressure at point A of the cap
(ten)
Absolute oil pressure at point B of the cap
(eleven)
Buy and download a ready-made solution.
Product Content: task solution in Word format.
No feedback yet