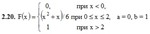DHS 18.2 - Option 20. Decisions Ryabushko AP
Content: 20v-IDZ18.2.doc (180.50 KB)
Uploaded: 10.11.2016
Positive responses: 0
Negative responses: 0
Sold: 2
Refunds: 0
$1.22
1. Find the distribution of the said discrete CB X and its distribution function F (x). Calculate the expectation M (X), the variance of D (X) and standard deviation σ (X). Draw the graph of the distribution function F (x)
1.20. The probability of winning the lottery with one ticket equals 1/6; SW X - the number of winning tickets of four.
2. Dana distribution function F (x) DM X. Find the probability density function f (x), the expectation M (X), the variance of D (X), and the probability of hitting NE X on the interval [a; b]. Construct the graphs of the functions F (x) and f (x).
3. Solve the following problems.
3.20. The number of fighters attacks, which may be subject to a bomber over the territory of the enemy, is a random variable distributed by the Poisson law with expectation a = 3. Each attack with probability 0.4 ends in defeat bomber. Determine the probability of hitting the bomber in the three attacks.
4. Solve the following problems.
4.20. To determine the average yield of a field of 10 000 hectares is expected to take a sample for one square meter per hectare in the area and to accurately estimate yields with these square meters. Rate the likelihood that the sample average yield will be different from the true average yield of the whole array is not more than 0.1 p, assuming that the standard deviation of the yield is less than 3 u?
1.20. The probability of winning the lottery with one ticket equals 1/6; SW X - the number of winning tickets of four.
2. Dana distribution function F (x) DM X. Find the probability density function f (x), the expectation M (X), the variance of D (X), and the probability of hitting NE X on the interval [a; b]. Construct the graphs of the functions F (x) and f (x).
3. Solve the following problems.
3.20. The number of fighters attacks, which may be subject to a bomber over the territory of the enemy, is a random variable distributed by the Poisson law with expectation a = 3. Each attack with probability 0.4 ends in defeat bomber. Determine the probability of hitting the bomber in the three attacks.
4. Solve the following problems.
4.20. To determine the average yield of a field of 10 000 hectares is expected to take a sample for one square meter per hectare in the area and to accurately estimate yields with these square meters. Rate the likelihood that the sample average yield will be different from the true average yield of the whole array is not more than 0.1 p, assuming that the standard deviation of the yield is less than 3 u?
Detailed solution. Decorated in Microsoft Word 2003 (Quest decided to use the formula editor)
For the convenience of viewing IDZ solutions on smartphones, an additional file in PDF format is sent
For the convenience of viewing IDZ solutions on smartphones, an additional file in PDF format is sent
No feedback yet